Bipolar Junction Transistor (BJT)- Biasing

Importance of BJT Biasing for Amplification

Definition:
- BJT biasing involves setting up a transistor amplifier for desired operating conditions.
Purpose:
- Establish the DC operating point.
- Ensure stability against variations like temperature changes.
Amplifier Analysis Components:
- DC Analysis: Determines steady-state operating point.
- AC Analysis: Determines response to input signals.
Superposition Principle: DC and AC analyses can be handled separately.
Energy Transfer:
- AC signal amplification is powered by energy from DC supplies.
Interdependence:
- DC operating point affects AC response and vice versa.

Biasing and its Significance

Biasing: Application of DC voltages to establish a fixed current and voltage level.
Q-Point (Quiescent Point): The operating point on device characteristics where the system is stable and inactive without a signal.
Purpose: Enables the transistor to amplify input signals effectively.
Real-World Use Cases:
- Audio amplifiers
- RF circuits
- Signal processing applications

Common Biasing Techniques

Fixed-Bias
Emitter-Bias
Voltage-Divider Bias
Collector Feedback
Emitter Follower
Common-Base

Revision of Important Facts

Basic Equations:
- \(V_{BE} \approx 0.7 \, \text{V}\)
- \(I_E = (\beta + 1) I_B \approx I_C\)
- \(I_C = \beta I_B\)
Base current \(I_B\) is usually determined first.
Key Biasing Requirements for Amplification:
1. Forward bias for base-emitter junction.
2. Reverse bias for base-collector junction.
3. Maintain operation in the active region for effective signal processing.
Impact of Temperature:
- Increases leakage current \(I_{CEO}\).
- Alters current gain \(\beta_{ac}\).
Stability Factor \(S\):
- Measures the effect of temperature on the Q-point stability.
- Higher stability is desirable.

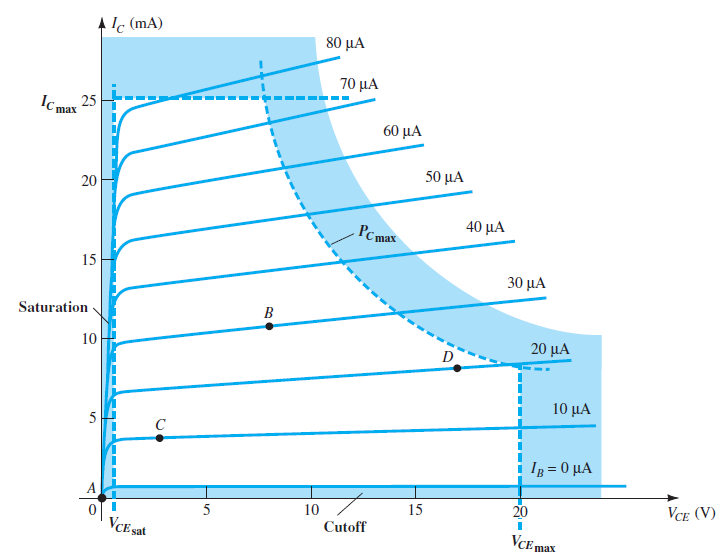
Q-Point Analysis

Operating Points:
- Point A: Device completely OFF (unsuitable for amplification).
- Point B: Ideal for small-signal amplification due to linear response.
- Point C: Limited swing and nonlinear response.
- Point D: High voltage operation, limiting positive voltage swing.
- Importance of Point B for Amplification
  - Ensures uniform amplification over signal swing.
  - Maintains consistent device gain.
  - Maximized at Point B without crossing into cutoff or saturation.

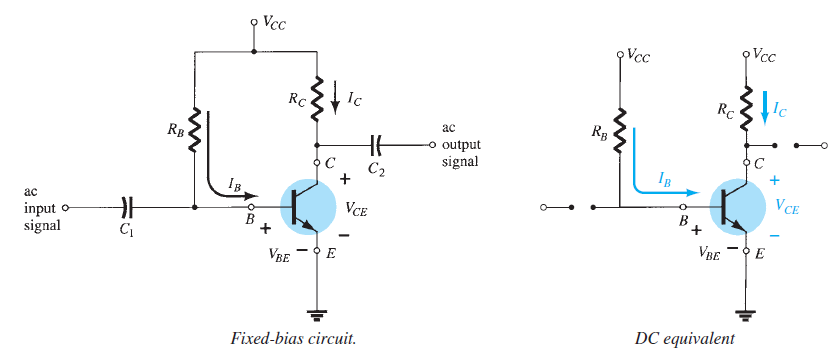
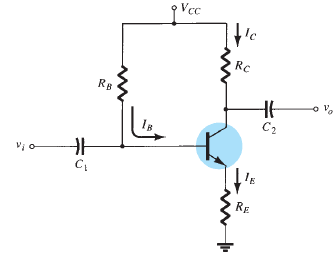
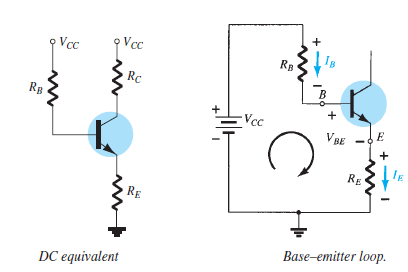
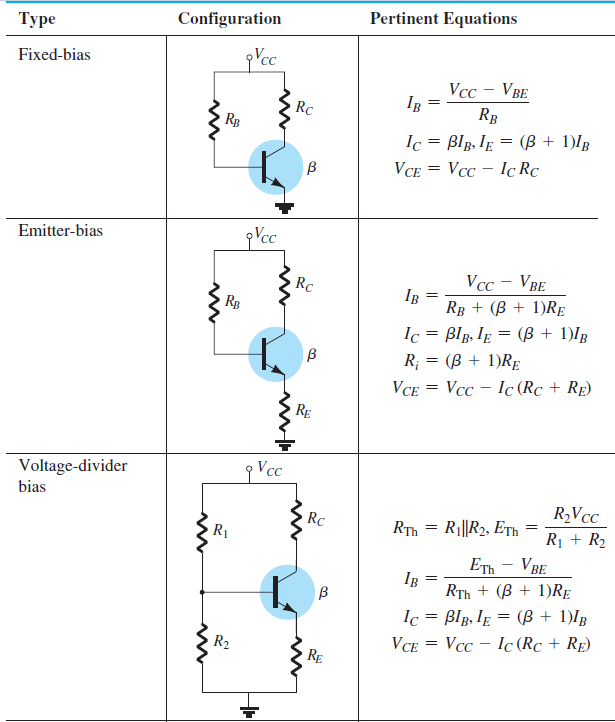
Fixed-Bias Configuration

Simplest DC bias configuration for a transistor.
Can be applied to both NPN and PNP transistors by changing current directions and voltage polarities.
For DC Analysis AC sources are removed by replacing capacitors with open circuits.
Supply \(V_{CC}\) is split into input and output sections to simplify analysis. \[X_C = \frac{1}{2\pi f C}\] At DC (\(f = 0\)), \(X_C = \infty \, \Omega\).

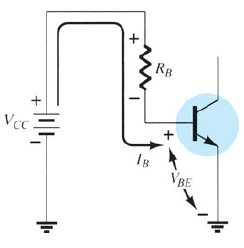
Base-Emitter Loop Analysis

Kirchhoff’s Voltage Law (KVL): \[+V_{CC} - I_B R_B - V_{BE} = 0\]
Base Current Equation: \[I_B = \frac{V_{CC} - V_{BE}}{R_B}\]
Key Insight: \(I_B\) is determined by \(R_B\) selection.

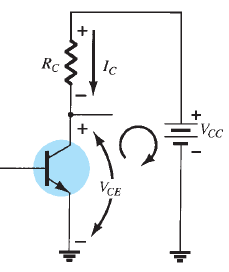
Collector-Emitter Loop Analysis

Current Relationship: \[I_C = \beta I_B\]
KVL Application: \[V_{CE} + I_C R_C - V_{CC} = 0 \quad \Rightarrow \quad V_{CE} = V_{CC} - I_C R_C\]
Observations: \(I_C\) is independent of \(R_C\) as long as the device remains in the active region. \(R_C\) affects the voltage \(V_{CE}\).

Important Relationships

Voltage Definitions: \[V_{CE} = V_C - V_E = V_C\] \[V_{BE} = V_B - V_E = V_B\]
Implications: \(V_E\) is often assumed as zero for simplicity. Control over \(V_{CE}\) is critical for setting the operating region.

Key Characteristics of Fixed-Bias Configuration

Simple to design and implement.
Provides control over base current through \(R_B\).
Sensitive to variations in \(\beta\) and temperature changes.
Primarily suited for low-power or stable environments.

Limitations and Stability Concerns

High sensitivity to temperature variations and transistor parameters.
Lack of automatic stabilization makes it less suitable for high-precision applications.
Improved configurations like voltage-divider bias address these concerns.

What is Saturation in Transistors?

Definition:
- A system is in saturation when it operates at maximum levels.
- Similar to a sponge fully soaked with water, unable to hold more.
In Transistors:
- Current reaches a maximum for the design.
- \(V_{CE}\) approaches or equals \(V_{CE_{\text{sat}}}\).
- Base–collector junction no longer reverse-biased, leading to distortion.

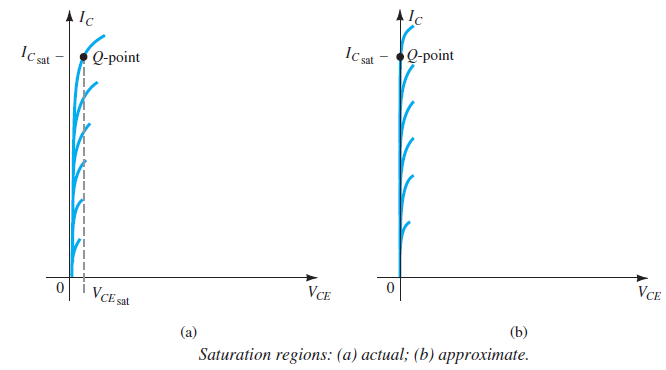
Saturation Characteristics

\(V_{CE} \approx 0~\mathrm{V}\)
Collector current at maximum \(I_{C_{\text{sat}}}\).
High \(I_C\) and low voltage across collector-emitter terminals.
Point where characteristic curves converge (Fig.).
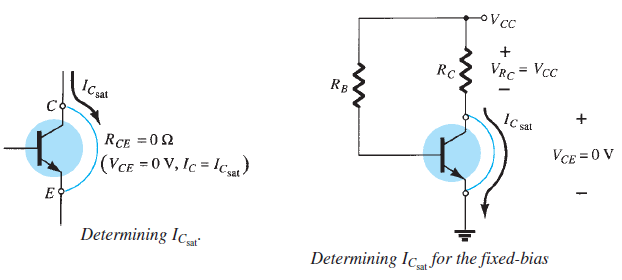
Treat the collector-emitter path as a short circuit.
Calculate the resulting \(I_C\) by assuming \(V_{CE} = 0~\mathrm{V}\).

\[R_{CE} = \frac{V_{CE}}{I_C} = \frac{0~\mathrm{V}}{I_{C_{\text{sat}}}} = 0~\Omega\]

Determining Saturation Current

Apply a short circuit between collector and emitter.
Voltage across \(R_C\) becomes \(V_{CC}\).

\[I_{C_{\text{sat}}} = \frac{V_{CC}}{R_C}\]
\(I_C\) should remain below \(I_{C_{\text{sat}}}\) for linear amplification.

Importance of Avoiding Saturation

Distortion Risk:
- Saturation disrupts linear amplification, introducing signal distortion.
Design Considerations:
- Ensure \(I_C\) operates well below \(I_{C_{\text{sat}}}\) for clean amplification.
Practical Applications:
- Avoiding saturation ensures consistent and accurate signal amplification.

Emitter-Bias Configuration

Objective: Improve stability over fixed-bias configuration.
Key Features:
- Addition of emitter resistor \(R_E\).
- Greater resistance to temperature variations and parameter shifts.
Application: Used for circuits requiring consistent performance under varying conditions.
\(R_E\) introduces feedback that stabilizes the circuit.
\(R_E\) impacts both base and collector-emitter loops.

Base–Emitter Loop Analysis

\[\begin{aligned} & +V_{CC} - I_B R_B - V_{BE} - I_E R_E = 0\\ & I_E = (\beta + 1) I_B \\ & I_B = \frac{V_{CC} - V_{BE}}{R_B + (\beta + 1) R_E} \end{aligned}\]

Compared to Fixed-Bias the additional term \((\beta + 1) R_E\) in the denominator improves stability.

Equivalent Circuit and Reflection Concept

\(R_E\) appears in base circuit as \((\beta + 1) R_E\) due to reflection effect.
Significantly larger apparent resistance due to \(\beta\) (50 or more).

\[\text{Input Resistance}~R_i = (\beta + 1) R_E\]
Greater \(R_i\) offers better temperature stability and reduces sensitivity to variations in \(\beta\).

Collector–Emitter Loop Analysis

\[\begin{aligned} & + I_E R_E + V_{CE} + I_C R_C - V_{CC} = 0 \\ & I_E \approx I_C \\ & V_{CE} = V_{CC} - I_C(R_C + R_E) \end{aligned}\]

\[\begin{aligned} & V_E = I_E R_E \\ & V_C = V_{CE} + V_E \quad \text{or} \quad V_C = V_{CC} - I_C R_C \\ & V_B = V_{CC} - I_B R_B \quad \text{or} \quad V_B = V_{BE} + V_E \end{aligned}\]

Voltage Levels and Biasing Points

Maintaining \(V_{CE}\) above saturation voltage ensures linear operation.
Proper selection of \(R_E\) and \(R_C\) determines \(V_{CE}\) and enhances stability.
Stabilizes operating point by providing negative feedback.
Ensures consistent operation despite changes in temperature or transistor parameters.

Voltage-Divider Bias

Biasing in BJTs sets the operating point (Q-point) for consistent performance.
Previous configurations depend on transistor’s current gain (\(\beta\)).
\(\beta\) is temperature-sensitive and varies, especially in silicon transistors.
Desire for bias circuits less dependent on \(\beta\).
Voltage-divider bias provides stable operating points despite \(\beta\) variations.
Proper circuit parameters lead to \(\beta\) independence for \(I_{CQ}\) and \(V_{CEQ}\).

Circuit Configuration

Resistors \(R_1\) and \(R_2\) form a voltage divider.
Bias point defined by \(I_{CQ}\) and \(V_{CEQ}\) stays stable.
Despite changes in \(\beta\), \(I_{CQ}\) and \(V_{CEQ}\) can remain fixed.

Analysis

\[\begin{aligned} R_{TH} & = R_1 || R_2 \qquad E_{TH} = V_{R_2} = \dfrac{R_2V_{CC}}{R_1+R_2} \\ &E_{TH} -I_BR_{TH}-V_{BE}-I_ER_E=0 \\ I_E &=(\beta+1)I_B,~\text{we get}\\ I_B & = \dfrac{E_{TH}-V_{BE}}{R_{TH}+(\beta+1)R_E} \\ V_{CE} & = V_{CC}-I_C(R_C+R_E) \\ \end{aligned}\]

\(V_E\), \(V_C\), and \(V_ B\) are same as obtained for the emitter-bias.

Benefits of Voltage-Divider Bias

Minimizes temperature and \(\beta\) sensitivity.
Stable Q-point ensures consistent circuit performance.
Versatile and widely used in practical designs.

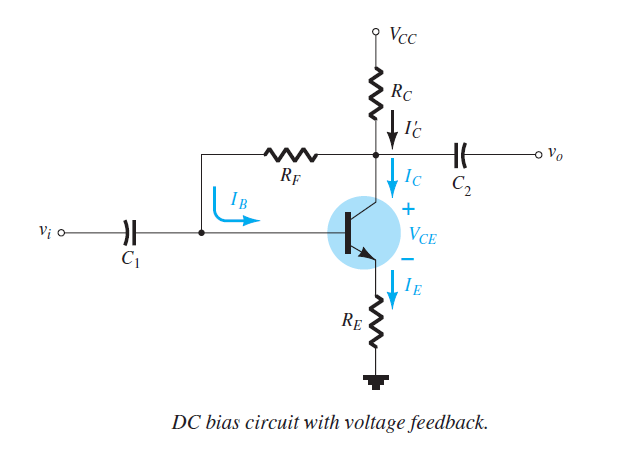
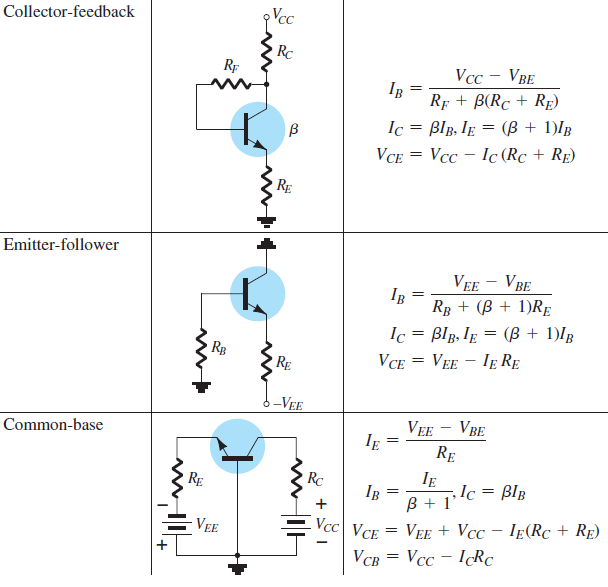
Collector Feedback Configuration

An improved level of stability by introducing a feedback path from collector to base
The Q-point is less sensitive to \(\beta\) and temperature changes than other biasing methods.

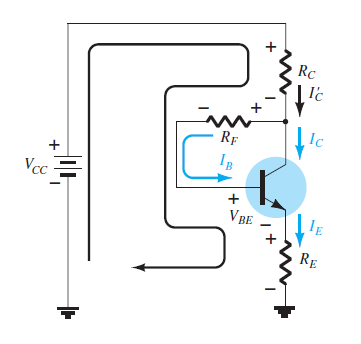
Base–Emitter Loop:

\[\begin{aligned} \text{KVL}~& V_{CC} - I_{C}^{\prime}R_C - I_BR_F - V_{BE} - I_ER_E =0 \\ &I_{C}^{\prime} = I_{C} + I_{B} \\ \text{Normally} ~& I_{C}^{\prime} \cong I_{C} = \beta I_B \quad I_E \cong I_{C} \\ \therefore ~ & V_{CC} - \beta I_B R_C - I_BR_F - V_{BE} - \beta I_B R_E =0 \\ \Rightarrow ~& I_B = \dfrac{V_{CC} - V_{BE} }{R_F + \beta (R_C + R_E)} \end{aligned}\]

In general, \[I_B = \dfrac{V^\prime}{R_F + \beta R^{\prime}}\]
For fixed-bias configuration \(\Rightarrow~\beta R^\prime\) does not exist
For the emitter-bias \(\Rightarrow~\beta+1 \cong \beta \qquad R^\prime = R_E\)
Because \(I_C = \beta I_B\), \[\begin{aligned} I_{CQ} & = \dfrac{\beta V^{\prime}}{R_F + \beta R^{\prime}} = \dfrac{V^\prime}{\dfrac{R_F}{\beta}+R^{\prime}} \\ &\cong \dfrac{V^\prime}{R^\prime}~ \left(\because R^\prime >> \dfrac{R_F}{\beta} \right) \\ \Rightarrow ~& \text{Independent of } \beta ~\text{variation} \end{aligned}\]

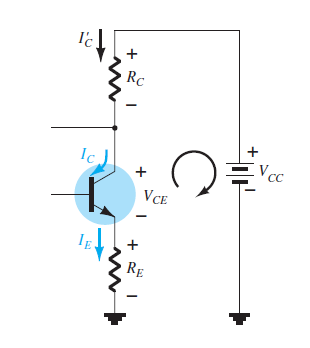
Collector–Emitter Loop:

\[\begin{aligned} \text{KVL}~& I_ER_E + V_{CE} + I_C^{\prime}R_C - V_{CC} =0 \\ \Rightarrow~& I_C (R_C + R_E) + V_{CE} - V_{CC} = 0 ~(\because ~I_C^\prime \cong I_C \quad I_E \cong I_C ) \\ \Rightarrow~& V_{CE} = V_{CC} - I_C (R_C + R_E)\\ \Rightarrow~& \text{Exactly same as emitter-bias and voltage-divider bias} \end{aligned}\]

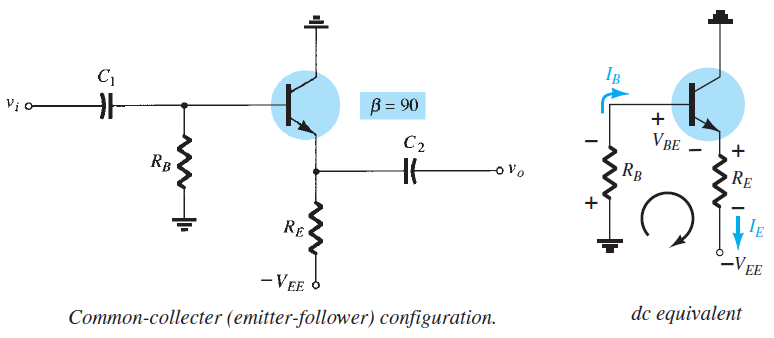
EMITTER-FOLLOWER CONFIGURATION

In collector-feedback, \(V_0\) is taken from collector terminal.
In emitter-follower, the output is taken off the emitter terminal.

\[\begin{aligned} \text{KVL}\quad & -I_BR_B - V_{BE} -I_ER_E + V_{EE} = 0\\ \text{Using} \quad & I_E = (\beta+1)I_B \\ \Rightarrow~& I_BR_B + (\beta+1)I_BR_E = V_{EE} - V_{BE} \\ \Rightarrow~& I_B = \dfrac{V_{EE} - V_{BE}}{R_B + (\beta+1)R_E} \end{aligned}\]
Output network: \[V_{CE} = V_{EE} - I_E R_E\]

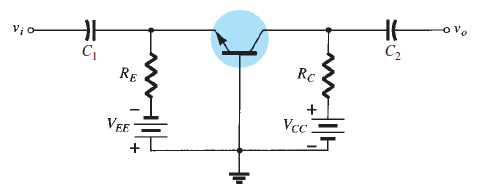
COMMON-BASE CONFIGURATION

Input signal is connected to the emitter terminal.
The base is held at ground or just above ground potential.
The common-base configuration is unique due to the use of two supplies and its design where the base is the common terminal.
Advantages: low input impedance, high output impedance, and good gain.

Input dc equivalent: \[\begin{aligned} & -V_{EE}+I_ER_E+V_{BE}=0 \\ & I_E=\frac{V_{EE}-V_{BE}}{R_E} \end{aligned}\]
Determining \(V_{CE}\) and \(V_{CB}\):

\[\begin{aligned} &-V_{EE}+I_{E}R_{E}+V_{CE}+I_{C}R_{C}-V_{CC}=0\\ &I_{E}\cong I_{C} \\ &\boxed{V_{CE}=V_{EE}+V_{CC}-I_E(R_C+R_E)} \\ V_{CB}&=V_{CC}-I_{C}R_{C}\\ I_{C}&\cong I_{E} \\ & \boxed{V_{CB}=V_{CC}-I_CR_C} \end{aligned}\]