Equivalent Circuit
Practical transformer excluding no-load current:
\(R_i\) and \(X_i\) represents resistance and leakage reactance
Practical transformer including no-load current components:
\(I_1 = I_0 + I_2^{\prime}\)
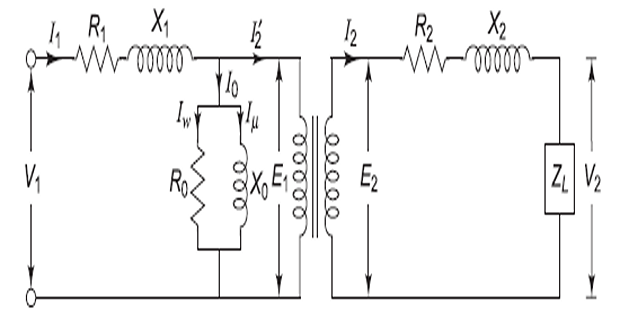
Practical transformer excluding no-load current:
\(R_i\) and \(X_i\) represents resistance and leakage reactance
Practical transformer including no-load current components:
\(I_1 = I_0 + I_2^{\prime}\)
Modified Circuit of Primary Winding:
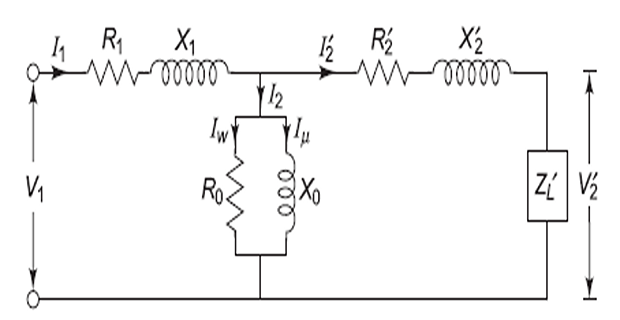\[\begin{aligned}
I_1^2R_2'& =I_2^2R_2 ~ \Leftarrow~\text{same power loss} \\
\Rightarrow~R_2'&
=\left(\frac{I_2}{I_1}\right)^2R_2=\frac{R_2}{K^2} \\
&\\
&\boxed{R_2' = \frac{R_2}{K^2}} ~\qquad
~\boxed{X_2' = \frac{X_2}{K^2}}
\end{aligned}\]
Note: Since all quantities are transferred to primary, the transformer need not be shown
As \(I_0\) is very small as compared to \(I_1~\Rightarrow\) drop across \(R_1\) and \(X_1\) due to \(I_0\) can be neglected \(\Rightarrow\) shift no-load components to the extreme left
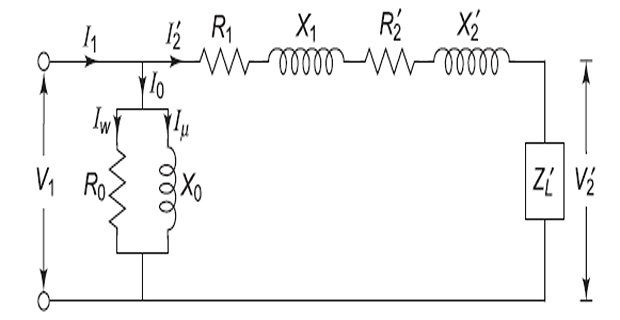Equivalent parameters referred to
primary:
\[\begin{aligned} R_{01} & =R_1+R_2'=R_1+\dfrac{R_2}{K^2} \\ X_{01} & =X_1+X_2'=X_1+\dfrac{X_2}{K^2} \\ Z_{01} & =\sqrt{R_{01}^2+X_{01}^2} \end{aligned}\]
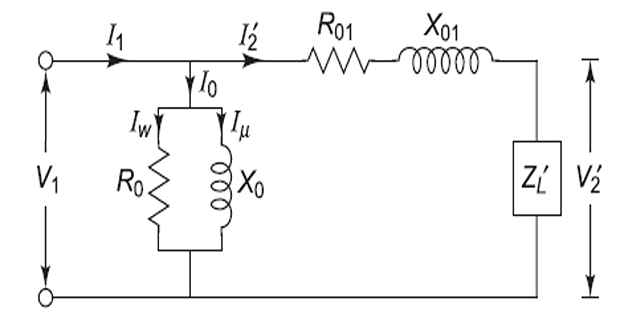
Equivalent parameters
referred to secondary:
\[\begin{aligned} R_{02} & =R_2+R_1'=R_2+K^2 \cdot R_1 \\ X_{02} & =X_2+X_1'=X_2+ K^2 \cdot X_1 \\ Z_{02} & =\sqrt{R_{02}^2+X_{02}^2} = K^2 \cdot Z_{01} \end{aligned}\]
Remember
Shifting primary R/X to the secondary, multiply it by \(K^2\)
Shifting secondary R/X to the primary, divide it \(K^2\)
Transformer loaded, \(V_2~\downarrow\) due to a drop across \(R_2\) and \(X_2\).
Change in \(V_2\) from no load to full load conditions, expressed as a fraction of the no-load secondary voltage is called regulation of the transformer. \[\begin{aligned} \text{Regulation} & =\frac{\left(\begin{matrix}\text{Secondary terminal}\\ \text{voltage on no load}\end{matrix}\right)-\left(\begin{matrix}\text{Secondary terminal}\\ \text{voltage on full load}\end{matrix}\right)}{\text{Secondary terminal voltage on no load}}\\ & = \dfrac{E_2-V_2}{E_2} \\ \text{%Regulation} & = \dfrac{E_2-V_2}{E_2} \times 100 \end{aligned}\]
\[\begin{aligned}
\text{Total voltage drop} & =E_2-V_2\\
&=O C-O A\\
&=O M-O A\\
&=A M=A N+N M\\
\end{aligned}\]
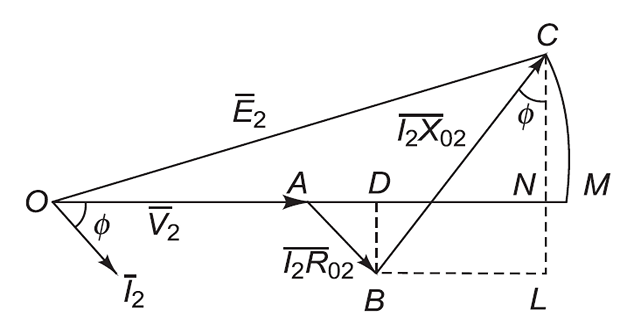
\[\begin{aligned} \text { Approximate voltage drop } & \approx A N \quad(\because N M \text { is very small) } \\ & =A D+D N \\ & =A D+B L \\ & =I_2 R_{02} \cos \phi+I_2 X_{02} \sin \phi \\ \% \text { regulation } & =\frac{I_2 R_{02} \cos \phi+I_2 X_{02} \sin \phi}{E_2} \times 100 \end{aligned}\] For leading pf, Approximate voltage drop \(=I_2 R_{02} \cos \phi-I_2 X_{02} \sin \phi\) \[\% \text { regulation }=\frac{I_2 R_{02} \cos \phi-I_2 X_{02} \sin \phi}{E_2} \times 100\]
\[\begin{aligned} &\boxed{\% \text { regulation } =\frac{I_2 R_{02} \cos \phi \pm I_2 X_{02} \sin \phi}{E_2} \times 100}\\ + &~~ \text{lagging pf} \qquad - ~~ \text{leading pf} \end{aligned}\]
\[\begin{aligned} \% {\color{red}{\textbf{regulation}}} & =\frac{I_1 R_{01} \cos \phi \pm I_1 X_{01} \sin \phi}{V_1} \times 100 \quad \Leftarrow~\text{primary side}\\ & \\ \% \text { regulation } & =\frac{100 I_2 R_{02}}{E_2} \cos \phi \pm \frac{100 I_2 X_{02}}{E_2} \sin \phi \\ & =\boxed{v_r \cos \phi \pm v_x \sin \phi }\\ &\boxed{v_r =\frac{ I_2 R_{02}}{E_2}\times 100}= {\color{magenta}{\textbf{percentage resistive drop }}}\\ & \boxed{v_x =\frac{I_2 X_{02}}{E_2}\times 100}= {\color{blue}{\textbf{percentage reactive drop }}} \end{aligned}\]
\[\begin{aligned} \eta & =\dfrac{\mbox{output power}}{\mbox{input power}}\\ &=\dfrac{\mbox{output power}}{\mbox{output power+losses}}\\ & =\dfrac{\mbox{output power}}{\mbox{output power+iron losses+copper losses}}\\ & \boxed{=\dfrac{V_{2}I_{2}\cos\Phi_{2}}{V_{2}I_{2}\cos\Phi_{2}+P_{i}+P_{c}}} \end{aligned}\] where \[\begin{array}{cc} V_{2} & \mbox{Secondary terminal voltage}\\ I_{2} & \mbox{Full-load secondary current}\\ \cos\Phi_{2} & \mbox{Power factor of the load}\\ P_{i} & \mbox{Iron losses =hysterises +eddy current losses}\\ P_{c} & \mbox{Full load copper losses = }I^{2}R_{T} \end{array}\]
If \(x\) is the fraction of the full-load, then efficiency is given as
\[\begin{aligned} \eta_{x} & =\boxed{\dfrac{x\times\mbox{Output}}{x\times\mbox{Output}+P_{i}+x^{2}P_{c}}}\\ &=\boxed{\dfrac{xV_{2}I_{2}\cos\Phi_{2}}{xV_{2}I_{2}\cos\Phi_{2}+P_{i}+x^{2}I_{2}^{2}R_{T}}} \end{aligned}\]
Condition for the maximum efficiency
\[\eta = \dfrac{V_2I_2\cos\Phi_2}{V_2I_2\cos\Phi_2+P_i+I_2^2R_T} = \dfrac{V_2\cos\Phi_2}{V_2\cos\Phi_2+P_i/I_2+I_2R_T}\]
\(V_2\) is constant
for a given \(\cos\Phi_2\), \(\eta\) depends upon \(I_2\)
efficiency will be maximum when \[\begin{aligned} \dfrac{d}{dI_{2}} & =\left(V_{2}\cos\Phi_{2}+\dfrac{P_{i}}{I_{2}}+I_{2}R_{T}\right)=0\\ & \Rightarrow0-\dfrac{P_{i}}{I_{2}^{2}}+R_{T}=0\\ & \Rightarrow P_{i}=I_{2}^{2}R_{T}\\ & \boxed{\Rightarrow\mbox{Iron loss}=\mbox{copper loss}} \end{aligned}\]
Value of output current for maximum efficiency \[I_2 = \sqrt{\dfrac{P_i}{R_T}}\] If \(x\) is the fraction of full load KVA at which \(\eta_{max}\). Then \[\boxed{ \mbox{Iron loss} =P_{i}}\qquad \boxed{\mbox{Copper loss} =x^{2}P_{c}}\] For maximum efficiency \(x^2P_c = P_i\) \[\boxed{x = \sqrt{\dfrac{P_i}{P_c}}}\] The output KVA corresponding to maximum efficiency \[\begin{aligned} \eta_{max} & =x\times KVA_{FL}\\ &\boxed{\Rightarrow\eta_{max} =KVA_{FL}\times\sqrt{\dfrac{P_{i}}{P_{c}}}} \end{aligned}\]
Open-Circuit Test (OC)
Purpose: To determine
iron loss or core loss (\(W_i\))
magnetising resistance (\(R_0\))
magnetising reactance (\(X_0\))
Test Connections:
HV open and supply and meters are connected to LV side
ammeter indicates no-load current (3-5% of F.L current)
Cu losses negligible and wattmeter indicates iron loss
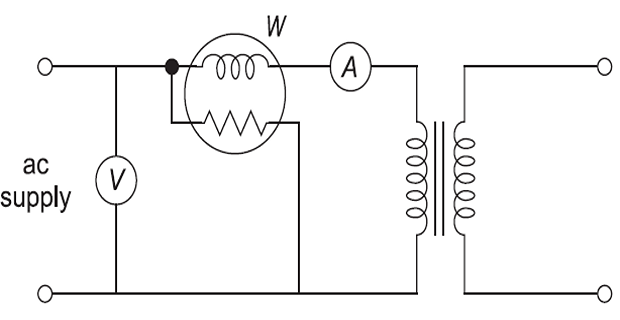
Calculation: \[\begin{aligned} & \text { Wattmeter reading }=W_i \\ & \text { Voltmeter reading }=V_1 \\ & \text { Ammeter reading }=I_0 \\ & W_i=V_1 I_0 \cos \phi_0 \\ & \boxed{\cos \phi_0=\frac{W_i}{V_1 I_0}} \\ & \boxed{I_w=I_0 \cos \phi_0} \qquad \boxed{I_\mu=I_0 \sin \phi_0} \\ & \boxed{R_0=\frac{V_1}{I_w}}\\ & \boxed{X_0=\frac{V_1}{I_\mu}} \\ & \end{aligned}\]
Short-Cicuit Test (SC)
Purpose: To determine
full-load copper loss
equivalent resistance (\(R_{01}\) or \(R_{02}\))
equivalent reactance (\(X_{01}\) or \(X_{02}\))
Test Connections:
LV side short-circuited while low-voltage is applied to other winding
Applied voltage slowly increased until F.L. current flows in the winding
Normally, the applied voltage is 5 to 10% of the rated voltage
Flux produced in core small & iron losses are very small.
Thus, wattmeter indicates full-load copper loss.
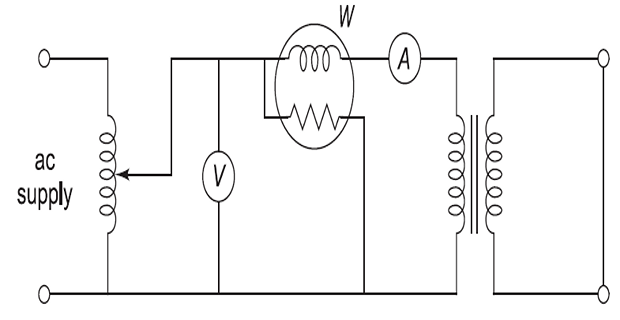
\[\begin{aligned} & \text { Wattmeter reading }=W_{\mathrm{sc}} \\ & \text { Voltmeter reading }=V_{\mathrm{sc}} \\ & \text { Ammeter reading }=I_{\mathrm{sc}} \\ &\\ &\boxed{ Z_{sc}=\frac{V_{\mathrm{sc}}}{I_{\mathrm{sc}}}} \\ & \boxed{R_{sc}=\frac{W_{\mathrm{sc}}}{I_{\mathrm{sc}}^2}} \\ & \boxed{X_{sc}=\sqrt{\left(Z_{sc}\right)^2-\left(R_{sc}\right)^2}} \end{aligned}\]