Problem-4
Find the all-day efficiency of 500 KVA distribution transformer whose
copper loss and iron loss at full load are 4.5 kW and 3.5 kW
respectively. During a day of 24 hours, it is loaded as under:
A \(11000 / 230 \mathrm{~V}, 150 \mathrm{KVA}, 1\) -phase \(, 50-\mathrm{Hz}\) transformer has core loss of \(1.4 \mathrm{KW}\) and F.L. Cu loss of \(1.6 \mathrm{KW}\). Determine
The KVA load for max efficiency and value of max efficiency at unity power factor
The efficiency at half F.L 0.8 of leading
Load kVA corresponding to maximum efficiency is \[=\text { F.L. } k V A \times \sqrt{\frac{\text { Iron loss }}{\text { F.L. Cu loss }}}=250 \times \sqrt{\frac{1.6}{1.4}}=160 \mathrm{kVA}\]
Since Cu loss equals iron loss at maximum efficiency,
\[\begin{aligned} \text{total loss} &=1.4+1.4=2.8 \mathrm{~kW}\\ \text{output} & =160 \times 1=160 \mathrm{~kW} \\ \eta_{\max }&=160 / 162.8=0.982 \text { or } 98.2 \% \end{aligned}\]
Cu loss at half full-load \(=1.6 \times(1 / 2)^{2}=0.4 \mathrm{~kW}\)
Total loss \(=1.4+0.4=1.8 \mathrm{~kW}\)
Half F.L. output at \(0.8 \mathrm{p.f}=(150 / 2) \times 0.8=60 \mathrm{~kW}\)
Efficiency \[60 /(60+1.8)=0.97~ \text{or}~ 97 \%\]
A 20-KVA, \(440 / 220 \mathrm{~V}, 1\) -phase, \(50 \mathrm{~Hz}\) transformer has iron loss of \(324 \mathrm{~W}\). The Cu loss is found to be \(100 \mathrm{~W}\) when delivering half full load current. Determine
efficiency when delivering full-load current at 0.8 lagging pf and
the percent of full load when the efficiency will be maximum.
F.L. Cu loss \(=2^{2} \times 100=400 \mathrm{~W} ;\)
Iron loss \(=324 \mathrm{~W}\)
F.L. efficiency at \(0.8 \mathrm{p} . \mathrm{f} .=\dfrac{20 \times 0.8}{(20 \times 0.8)+0.724} \times 100=95.67 \%\)
\(\dfrac{\mathrm{kVA} \text { for maximum }}{\mathrm{F.L}, \mathrm{kVA}}=\sqrt{\dfrac{\text { Iron loss }}{\text { F.L. Cu loss }}}=\sqrt{\dfrac{324}{400}}=0.9\)
Hence, efficiency would be maximum at \(90 \%\) of F.L.
A 600 KVA, 1-phase transformer has an efficiency of 92% both at full-load and half-load at UPF. Determine its efficiency at 60% of full load at 0.8 power factor lag.
Efficiency: \[\eta=\frac{x \times k V A \times \cos \phi}{(x \times k V A) \times \cos \phi+W_{i}+x^{2} W_{C u}} \times 100\] where \(x\) represents percentage of full-load \(W_{i}\) is iron loss and \(W_{C u}\) is full-load Cu loss:
At FI. u.p.f. \(\quad\) Here \(x=1\)
\[\begin{aligned} 92&=\frac{1 \times 600 \times 1}{1 \times 600 \times 1+W_{i}+1^{2} W_{C u}} \times 100 \\ W_{i}+W_{Cu}&=52.174 \mathrm{~kW} \end{aligned}\]
At half F.L. UPF. Here \(x=1 / 2\) \[\begin{aligned} 92&=\frac{1 / 2 \times 600 \times 1}{(1 / 2) \times 600 \times 1+W_{i}+(1 / 2)^{2} W_{C u}} \times 100\\ W_{i}+0.25 W_{\mathrm{Cu}}&=26.087 \mathrm{~kW} \end{aligned}\]
From \((i)\) and \((i i),\) we get, \(W_{i}=17.39 \mathrm{~kW}, W_{C_{u}}=34.78 \mathrm{~kW}\)
\(60 \%\) F.L. 0.8 p.f. (lag) Here, \(x=0.6\) \[\eta=\frac{0.6 \times 600 \times 0.8 \times 100}{(0.6 \times 600 \times 0.8)+17.39+(0.6)^{2} 34.78}=85.9 \%\]
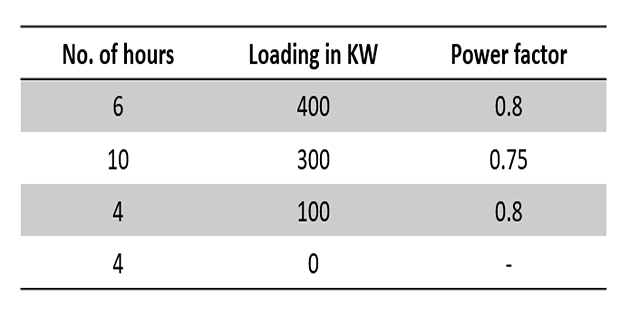
Find the all-day efficiency of 500 KVA distribution transformer whose
copper loss and iron loss at full load are 4.5 kW and 3.5 kW
respectively. During a day of 24 hours, it is loaded as under:

A load of \(400 \mathrm{~kW}\) at 0.8 p.f. is equal to \(400 / 0.8=500 \mathrm{kVA}\).
\(300 \mathrm{~kW}\) at 0.75 p.f. means \(300 / 0.75=400 \mathrm{kVA}\)
\(100 \mathrm{~kW}\) at 0.8 p.f. means \(100 / 0.8=125 \mathrm{kVA}\) i.e., one-fourth of the full-load. \[\begin{aligned} \text { Cu loss at } F . L \text { of } 500 \mathrm{kVA} &=4.5 \mathrm{~kW} \\ \text { Cu loss at } 400 \mathrm{kVA} &=4.5 \times(400 / 500)^{2}=2.88 \mathrm{~kW} \\ \text { Cu loss at } 125 \mathrm{kVA} &=4.5 \times(125 / 500)^{2}=0.281 \mathrm{~kW} \end{aligned}\]
Total Cu loss in 24 hours \[\begin{aligned} &=(6 \times 4.5)+(10 \times 2.88)+(4 \times 0.281)+(4 \times 0)\\ &=56.924 \mathrm{kWh} \end{aligned}\]
The iron loss takes place throughout the day irrespective of the load on the transformer because its primary is energized all the 24 hours.
Iron loss in 24 hours \(=24 \times 3.5=84 \mathrm{kWh}\)
Total transformer loss \(=56.924+84=140.924 \mathrm{kWh}\)
Transformer output in 24 hours \(=(6 \times 400)+(10 \times 300)+(4 \times 100)=5800 \mathrm{kWh}\)
All-day efficiency \[\begin{aligned} \eta_{\text {all-day }} &=\frac{\text { output }}{\text { output }+\operatorname{losses}}\\ &=\frac{5800}{5800+140.924}=0.976 \\ &=97.6 \% \end{aligned}\]