The entire power flow in the motor is given below.
FL. efficiency \[\begin{aligned} &=100,000 / 108,995=0.917 & = 91.7 \% \end{aligned}\]
A 440-V, 3-phase, 50-Hz, Y-connected induction motor has a full-load speed of 1425 rpm. The rotor has an impedance of \((0.4+j4)~ \Omega\) and rotor/stator turn ratio of 0.8. Calculate
Full load-torque
Rotor current and full-load rotor Cu loss
Power output if windage and friction losses amount to 500 W
Maximum torque and the speed at which it occurs
Starting current
Starting torque
Synchronous speed: \(N_{s}=120 \times 50 / 4=1500 \mathrm{rpm}=25 \mathrm{rps}\)
Slip: \(s=75 / 1500=0.05\)
\(E_{1}=440 / 1.73=254 \mathrm{~V} / \mathrm{phase}\)
Full-load torque: \[\begin{aligned} T_{f}&=\frac{3}{2 \pi \times 25} \times \frac{0.05(0.8 \times 254)^{2} \times 0.4}{(0.4)^{2}+(0.05 \times 4)^{2}} \\ &=78.87 \mathrm{~N}-\mathrm{m} \end{aligned}\]
Rotor Current: \[\begin{aligned} I_{r} &=\frac{s E_{2}}{\sqrt{R_{2}^{2}+\left(s X_{2}\right)^{2}}}=\frac{s K E_{1}}{\sqrt{R_{2}^{2}+\left(s X_{2}\right)^{2}}}=\frac{0.05 \times(0.8 \times 254)}{\sqrt{(0.4)^{2}+(0.05 \times 4)^{2}}} \\ &=22.73 \mathrm{~A} \end{aligned}\]
Total Cu loss: \[=3 I_{r}^{2} R=3 \times 22.73^{2} \times 0.4=620 \mathrm{~W}\]
Now; \[\begin{aligned} P_{m} &=2 \pi N T=2 \pi \times(1425 / 60) \times 78.87=11,745 \Omega \\ P_{\text {out }} &=P_{m}-\text { Windage and friction loss }\\ &=11,745-500=11,245 \mathrm{~W} \end{aligned}\]
For maximum torque, \(\quad s=R_{2} / X_{2}=0.4 / 4=0.1\)
Maximum torque: \[T_{\max }=\frac{3}{2 \pi \times 25} \times \frac{0.1 \times(0.8 \times 254)^{2} \times 0.4}{(0.4)^{2}+(0.1 \times 4)^{2}}=98.5 \mathrm{~N}-\mathrm{m}\]
Since \(s=0.1\), slip speed \(=s N_{s}=0.1 \times 1500=150 \mathrm{rpm} .\)
Speed for maximum torque \(=1500-150=1350\) rpm.
Starting Current: \[=\frac{E_{2}}{\sqrt{R_{2}^{2}+X_{2}^{2}}}=\frac{K E_{1}}{\sqrt{R_{2}^{2}+X_{2}^{2}}}=\frac{0.8 \times 254}{\sqrt{0.4^{2}+4^{2}}}=50.5 \mathrm{~A}\]
Starting Torque: At start, s=1, hence \[T_{st}=\frac{3}{2 \pi \times 25} \times \frac{(0.8 \times 254)^{2} \times 0.4}{(0.4)^{2}+4^{2}}=19.5 \mathrm{~N}-\mathrm{m}\]
A 100-KW, 3300-V, 50-Hz, 3-phase, star connected induction motor has a synchronous speed of 500 rpm. The full-load slip is 1.8% and F.L power factor 0.85. Stator copper loss = 2440 W. Iron loss = 3500 W. Rotational losses = 1200 W. Calculate:
the rotor copper loss
the line current
the full-load efficiency
\(P_{m}= \text{output} +\text{rotational loss} =100+1.2=101.2 \mathrm{~kW}\)
Rotor Cu loss: \[=\frac{s}{1-s} \times P_{m}=\frac{0.018}{1-0.018} \times 101.2=1.855 \mathrm{~kW}\]
Rotor input power: \[P_{2} =P_{m}+\text { rotor } \mathrm{Cu} \text { loss }=101.2+1.855=103.055 \mathrm{~kW}\]
Stator input power: \[\begin{aligned} &=P_{2}+\text { stator } \mathrm{Cu} \text { and iron losses } \\ &=103.055+2.44+3.5=108.995 \mathrm{~kW} \end{aligned}\]
Line current: \[\begin{aligned} 108,995 &=\sqrt{3} \times 3300 \times I_{L} \times 0.85 \\ \Rightarrow~\mathrm{I}_{\mathrm{L}}&=22.4 \mathrm{~A} \end{aligned}\]
The entire power flow in the motor is given below.
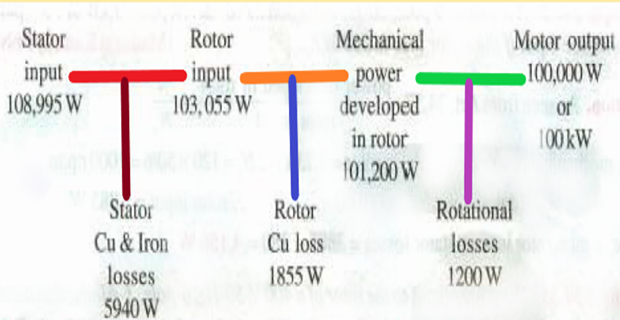
FL. efficiency \[\begin{aligned} &=100,000 / 108,995=0.917 & = 91.7 \% \end{aligned}\]