Efficiency of a transformer

\[\begin{aligned} \eta & =\dfrac{\mbox{output power}}{\mbox{input power}}=\dfrac{\mbox{output power}}{\mbox{output power+losses}}\\ & =\dfrac{\mbox{output power}}{\mbox{output power+iron losses+copper losses}}\\ & =\dfrac{V_{2}I_{2}\cos\Phi_{2}}{V_{2}I_{2}\cos\Phi_{2}+P_{i}+P_{c}} \end{aligned}\] where \[\begin{array}{cc} V_{2} & \mbox{Secondary terminal voltage}\\ I_{2} & \mbox{Full-load secondary current}\\ \cos\Phi_{2} & \mbox{Power factor of the load}\\ P_{i} & \mbox{Iron losses =hysterises +eddy current losses}\\ P_{c} & \mbox{Full load copper losses = }I^{2}R_{T} \end{array}\] If \(x\) is the fraction of the full-load, then efficiency is given as \[\eta_{x}=\dfrac{x\times\mbox{Output}}{x\times\mbox{Output}+P_{i}+x^{2}P_{c}}=\dfrac{xV_{2}I_{2}\cos\Phi_{2}}{xV_{2}I_{2}\cos\Phi_{2}+P_{i}+x^{2}I_{2}^{2}R_{T}}\]

Condition for the maximum efficiency

\[\eta = \dfrac{V_2I_2\cos\Phi_2}{V_2I_2\cos\Phi_2+P_i+I_2^2R_T} = \dfrac{V_2\cos\Phi_2}{V_2\cos\Phi_2+P_i/I_2+I_2R_T}\] Now, \(V_2\) is constant. Thus for a given \(\cos\Phi_2\), \(\eta\) depends upon \(I_2\). Hence, efficiency will be maximum when \[\begin{aligned} \dfrac{d}{dI_{2}} & =\left(V_{2}cos\Phi_{2}+\dfrac{P_{i}}{I_{2}}+I_{2}R_{T}\right)=0\\ & \Rightarrow0-\dfrac{P_{i}}{I_{2}^{2}}+R_{T}=0\\ & \Rightarrow P_{i}=I_{2}^{2}R_{T}\\ & \Rightarrow\mbox{Iron loss}=\mbox{copper loss} \end{aligned}\] Value of output current for maximum efficiency \[I_2 = \sqrt{\dfrac{P_i}{R_T}}\] If \(x\) is the fraction of full load KVA at which \(\eta_{max}\). Then \[\begin{aligned} \mbox{Iron loss} & =P_{i}\\ \mbox{Copper loss} & =x^{2}P_{c} \end{aligned}\] For maximum efficiency \(x^2P_c = P_i\) \[x = \sqrt{\dfrac{P_i}{P_c}}\] The output KVA corresponding to maximum efficiency \[\begin{aligned} \eta_{max} & =x\times KVA_{FL}\\ \Rightarrow\eta_{max} & =KVA_{FL}\times\sqrt{\dfrac{P_{i}}{P_{c}}} \end{aligned}\]

All-Day Efficiency

• Power transformer (at generating station) and Distribution transformer (distribution substation)

• Power transformers are switched in or out of the circuit depending upon the load to be handled

• Distribution transformer is never switched off remain irrespective of the load

• In such case, constant loss continues to be dissipated

• Concept of energy based efficiency arise - "all day efficiency"

  \[\begin{aligned} \%\mbox{all-day}\eta & =\dfrac{\mbox{Output energy in KWh during a day}}{\mbox{Input energy in KWh during a day}}\times100\\ & =\dfrac{\mbox{Output energy in KWh during a day}}{\mbox{Output energy +Energy spent for total losses}}\times100 \end{aligned}\]

Auto-Transformer

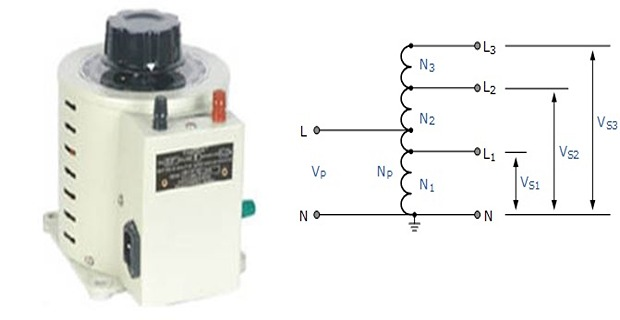
• Transformer with one winding only

• Part of the winding is common to both primary and secondary

• No electrical isolation as in 2-winding transformer

• Theory and operation remains similar

• Because of one winding, uses less copper and hence cheaper

• It is used where transformation ratio varies little from unity

  

  

  Saving of Cu

• Volume (or weight) \(\propto\) length and area of cross-section
  length of conductor \(\propto\) number of turns
  cross-section depends on current
  Weight \(\propto\) product of current and number of turns

  Wt. of Cu in section AC is \(\propto\) \((N_1-N_2)I_1\)
  Wt. of Cu in section BC is \(\propto\) \(N_2(I_2-I_1)\)
  \(\therefore\) Total Wt. of Cu \(\propto\) \((N_1-N_2)I_1+N_2(I_2-I_1)\)
  

• If a 2-winding T/F perform the same task, then
  Wt. of Cu in primary \(\propto\) \(N_1I_1\)
  Wt. of Cu in secondary \(\propto\) \(N_2I_2\)
  \(\therefore\) Total Wt. of Cu \(\propto\) \(N_1I_1+N_2I_2\) \[\therefore \dfrac{\mbox{Wt. of Cu in auto-transformer}}{\mbox{Wt. of Cu in ordinary transformer}} = \dfrac{(N_1-N_2)I_1+N_2(I_2-I_1)}{N_1I_1+N_2I_2} = (1-K)\]

  \(\Rightarrow\) \(W_a\) = \((1-K) \times W_0\)

  \[\boxed{\text{Saving}=W_0-W_a=W_0-(1-K)W_0=KW_0}\]

  Hence, saving will increase as \(K\) approaches unity
  \(\Rightarrow\) Power transferred inductively is

  \[\boxed{P_{ind}=Input\times(1-K)}\]

  \(\Rightarrow\) The rest of the power is conductively transferred

  \[\boxed{P_{cond}=\text{Input}\times K}\]

  Advantages of Auto transformer

  • Less costly

  • Better regulation

  • Low losses as compared to 2-winding T/F of the same rating

  Disadvantages of Auto transformer

  • The secondary winding is not insulated from primary so if a low supply voltage is used from a high voltage and if a break occurs in secondary winding then full primary voltage comes across the secondary terminal which is dangerous to the operator and the equipment.

  • used only in limited places where a slight variation of the output voltage from input voltage is required.

  Applications of Auto transformer

  • Used as a starter to give 50-60% of full voltage to the stator of a squirrel cage induction motor during starting

  • used to give a small boost to a distribution cable, to correct the voltage drop

  • used as a voltage regulator

  • in power T & D system and also in audio system and railways