Power System Load Characteristics and Utlization

Problem-1:

The maximum demand on a power station is \(100 \mathrm{MW}\). If the annual load factor is \(40 \%\), calculate the total energy generated in a year.

Solution-1

\[\begin{aligned} \text { Energy generated/year } &=\text { Max. demand } \times \text { L.F. } \times \text { Hours in a year } \\ &=\left(100 \times 10^{3}\right) \times(0.4) \times(24 \times 365) \mathrm{kWh} \\ &=3504 \times 10^{5} \mathrm{kWh} \end{aligned}\]

Problem-2:

A generating station has a connected load of \(43 \mathrm{MW}\) and a maximum demand of \(20 \mathrm{MW} ;\) the units generated being \(61.5 \times 10^{6}\) per anmum. Calculate the following:

1. the demand factor and

2. load factor:

Solution-2

• Demand factor \(=\dfrac{\text { Max. demand }}{\text { Connected load }}=\frac{20}{43}=0 \cdot 465\)

• Average demand \(=\dfrac{\text { Units generated / annum }}{\text { Hours in a year }}=\frac{61 \cdot 5 \times 10^{6}}{8760}=7020 \mathrm{kW}\)

• Load factor \(=\dfrac{\text { Average demand }}{\text { Max. demand }}=\dfrac{7020}{20 \times 10^{3}}=0.351\) or \(35 \cdot 1 \%\)

Problem-3:

A \(100 \mathrm{MW}\) power station delivers

1. \(100 \mathrm{MW}\) for 2 hours

2. \(50 \mathrm{MW}\) for 6 hours

3. is shut down for the rest of each day

4. It is also shut down for maintenance for 45 days each year

Calculate its annual load factor:

Solution-3

• Energy supplied for each working day \[=(100 \times 2)+(50 \times 6)=500 \mathrm{MWh}\]

• Station operates for \(=365-45=320\) days in a year

• Energy supplied/year \(=500 \times 320=160,000 \mathrm{MWh}\)

• Annual load factor \[\begin{aligned} &=\frac{\text { MWh supplied per annum }}{\text { Max. demand in MW } \times \text { Working hours }} \times 100 \\ &=\frac{160,000}{(100) \times(320 \times 24)} \times 100=20 \cdot 8 \% \end{aligned}\]

Problem-4:

A generating station has a maximum demand of \(25 \mathrm{MW}\), a load factor of \(60 \%\), plant capacity factor of \(50 \%\) and plant use factor of \(72 \%\).

Find

1. the reserve capacity of the plant

2. the daily energy produced and

3. maximum energy that could be produced daily if the plant while running as per schedule, were fully loaded.

Solution-4

• Load factor \(=\frac{\text { Average demand }}{\text { Maximum demand }}\)

  \[\begin{aligned} 0 \cdot 60 & =\frac{\text { Average demand }}{25} \\ \Rightarrow \text{Average demand} & =25 \times 0.60=15 \mathrm{MW} \end{aligned}\]

• Plant capacity factor \(=\frac{\text { Average demand }}{\text { Plant capacity }}\)

  \[\begin{aligned} \Rightarrow \text{ Plant capacity}& =\frac{\text { Average demand }}{\text { Plant capacity factor }}=\frac{15}{0 \cdot 5}=30 \mathrm{MW} \end{aligned}\]

• Reserve capacity of plant \(=\) Plant capacity - maximum demand \[=30-25=5 \mathrm{MW}\]

• Daily energy produced

  \[\begin{aligned} & = \text{Average demand} \times 24 \\ & =15 \times 24=360 \mathrm{MWh} \end{aligned}\]

• Maximum energy that could be produced

  \[\begin{aligned} & =\frac{\text { Actual energy produced in a day }}{\text { Plant use factor }} \\ & =\frac{360}{0.72}=500 ~ \mathrm{MWh} / \mathrm{day} \end{aligned}\]

Problem-5:

A diesel station supplies the following loads to various consumers:

If the maximum demand on the station is \(2500 ~\mathrm{kW}\) and the number of \(\mathrm{kWh}\) generated per year is \(45 \times 10^{5},\) determine

• the diversity factor

• annual load factor

Solution-5

• Diversity factor \(=\dfrac{1500+750+100+450}{2500}=1 \cdot 12\)

• Average demand \(=\dfrac{\text { kWh generated } / \text { annum }}{\text { Hours in a year }}=\dfrac{45 \times 10^{5}}{ 8760}=513 \cdot 7 \mathrm{kW}\)

• Load factor \(=\dfrac{\text { Average load }}{\text { Max. demand }}=\dfrac{513 \cdot 7}{2500}=0 \cdot 205=20 \cdot 5 \%\)

Problem-6:

A power station has

• a maximum demand of \(15000 \mathrm{kW}\)

• The annual load factor is 50%

• plant capacity factor is 40%.

Determine the reserve capacity of the plant.

Solution-6

• Energy generated/annum \[\begin{aligned} &=\text { Max. demand } \times \text { L.F. } \times \text { Hours in a year } \\ &=(15000) \times(0.5) \times(8760) \mathrm{kWh} \\ &=65.7 \times 10^{6} \mathrm{kWh} \end{aligned}\]

• Plant capacity factor \(=\dfrac{\text { Units generated } / \text { annum }}{\text { Plant capacity } \times \text { Hours in a year }}\) \[\begin{aligned} \Rightarrow \text{Plant capacity} &=\frac{65 \cdot 7 \times 10^{6}}{0 \cdot 4 \times 8760}=18,750 \mathrm{kW} \end{aligned}\]

• Reserve capacity \[\begin{aligned} &=\text { Plant capacity - Max. demand } \\ &=18,750-15000=3750 \mathrm{kW} \end{aligned}\]

Problem-7:

A power supply is having the following loads :

If the overall system diversity factor is \(1 \cdot 35,\) determine

1. maximum demand

2. connected load of each type.

Solution-7

• The sum of maximum demands of three types of loads is \(=1500+2000+10,000=13,500\) kW.

• As the system diversity factor is 1.35 , \(\Rightarrow\) Max. demand on supply system \(=13,500 / 1 \cdot 35=10,000 \mathrm{kW}\)

• Each type of load has its own diversity factor among its consumers. Sum of max. demands of different domestic consumers \(=\) Max. domestic demand \(\times\) diversity factor \[=1500 \times 1 \cdot 2=1800 \mathrm{kW}\]

• Connected domestic load \(=1800 / 0 \cdot 8=2250 \mathrm{kW}\)

• Connected commercial load \(=2000 \times 1 \cdot 1 / 0 \cdot 9=2444 \mathrm{kW}\)

• Connected industrial load \(=10,000 \times 1 \cdot 25 / 1=12,500 \mathrm{kW}\)

Problem-8:

At the end of a power distribution system, a certain feeder supplies three distribution transformers, each one supplying a group of customers whose connected loads are as under:

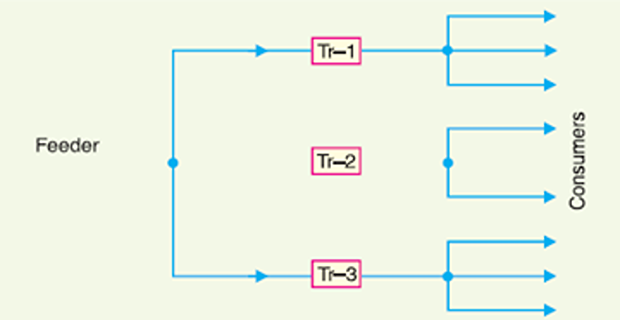
If the diversity factor among the transformers is \(1 \cdot 3,\) find the maximum load on the feeder.

Solution-8

• Sum of max. demands of customers on Transformer 1 \[=\text { connected load } \times \text { demand factor }=10 \times 0.65=6.5 \mathrm{kW}\]

• As the diversity factor among consumers connected to transformer No. 1 is \(1 \cdot 5\), \[\therefore \quad \text { Maximum demand on Transformer } 1=6.5 / 1 \cdot 5=4 \cdot 33 \mathrm{kW}\]

• Maximum demand on Transformer \(2=12 \times 0.6 / 3 \cdot 5=2.057 \mathrm{kW}\)

• Maximum demand on Transformer \(3=15 \times 0.7 / 1 \cdot 5=7 \mathrm{kW}\)

• As the diversity factor among transformers is \(1 \cdot 3\),

  \[\text{Maximum demand on feeder}=\frac{4 \cdot 33+2 \cdot 057+7}{1 \cdot 3}=10 \cdot 3 \mathrm{kW}\]

Problem-9:

It has been desired to install a diesel power station to supply power in a suburban area having the following particulars:

1. 1000 houses with average connected load of \(1 \cdot 5 \mathrm{kW}\) in each house. The demand factor and diversity factor being 0.4 and 2.5 respectively.

2. 10 factories having overall maximum demand of \(90 \mathrm{kW}\).

3. 7 tubewells of \(7 \mathrm{kW}\) each and operating together in the morning. The diversity factor among above three types of consumers is 1 - \(2 .\)

What should be the minimum capacity of power station ?

Solution-9

• Sum of max. demands of houses \(=(1 \cdot 5 \times 0 \cdot 4) \times 1000=600 \mathrm{kW}\)

• Max. demand for domestic load \(=600 / 2 \cdot 5=240 \mathrm{kW}\)

• Max. demand for factories \(=90 \mathrm{kW}\)

• Max. demand for tubewells \(=7^{*} \times 7=49 \mathrm{kW}\)

• The sum of maximum demands of three types of loads is \(=240+90+49=379 \mathrm{kW}\). As the diversity factor among the three types of loads is \(1 \cdot 2\),

• Max. demand on station \(=379 / 1 \cdot 2=316 \mathrm{kW}\)

• Minimum capacity of station requried \(=316 \mathrm{kW}\)

Problem-10:

A generating station has the following daily load cycle :

Draw the load curve and find

1. maximum demand

2. units generated per day

3. average load

4. load factor:

Solution-10

Daily curve is drawn by taking the load along \(Y\) -axis and time along \(X\) -axis. For the given load cycle, the load curve is shown

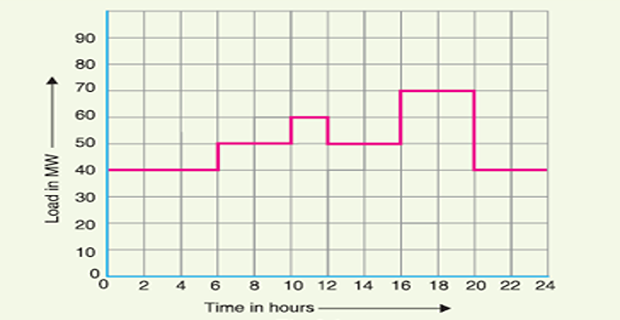
(i) It is clear from the load curve that maximum demand on the power station is \(70 \mathrm{MW}\) and occurs during the period \(16-20\) hours. \[\therefore \text { Maximum demand }=70~ \mathrm{MW}\]