Solved Problems on Maximum Power Transfer Theorem

Demonstrative Video

Problem-1

Determine the maximum power delivered to the resistor \(R\).

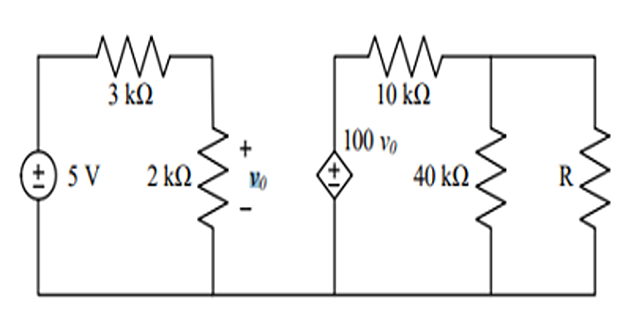
Solution-1

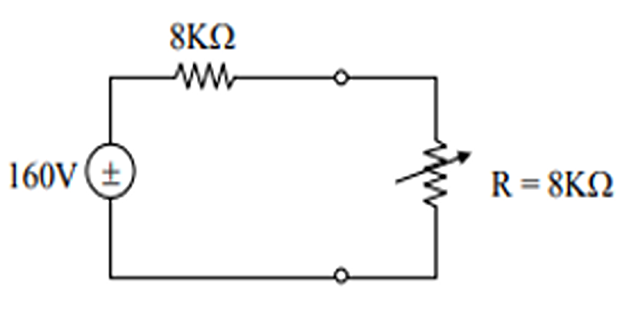
\[\begin{aligned} V_0 & = \dfrac{2 K\Omega}{5 K\Omega}(5) = 2~\mathrm{V} \\ V_{oc} & = V_{TH} = V_{40 K\Omega} = \dfrac{40K\Omega}{50K\Omega}(200)=160~\mathrm{V}\\ I_{sc} & = \dfrac{200}{10K\Omega} = 20~\mathrm{mA} \\ R_{th} & = \dfrac{V_{th}}{I_{sc}} = \dfrac{160\mathrm{V}}{20\mathrm{mA}}=8~K\Omega \\ P_{max} & = \dfrac{V_{th}^2}{4R_L} = \dfrac{160^2}{4\times 8K\Omega} = 0.8~\mathrm{W} \end{aligned}\]

Problem-2

Determine the value of \(R_L\) for maximum power transfer.

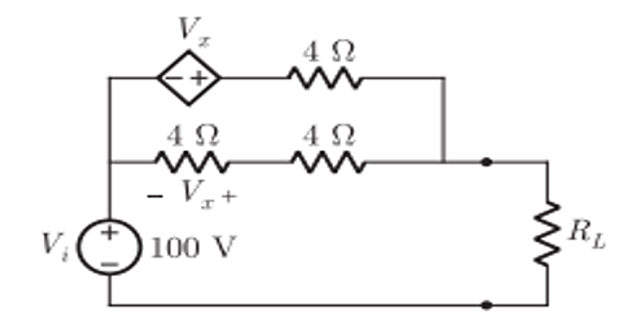
Solution-2

\[\begin{aligned} V_1 & = V_x +4\left(I_1-\dfrac{V_x}{4}\right)=4I_1\\ R_{th} & = \dfrac{V_1}{I_1} = 4~\Omega \end{aligned}\]

Problem-3

Find the value of R in the circuit such that maximum power transfer takes place. What is the amount of this power?

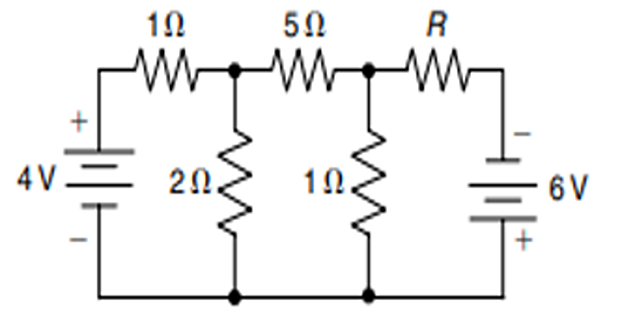
Solution-3

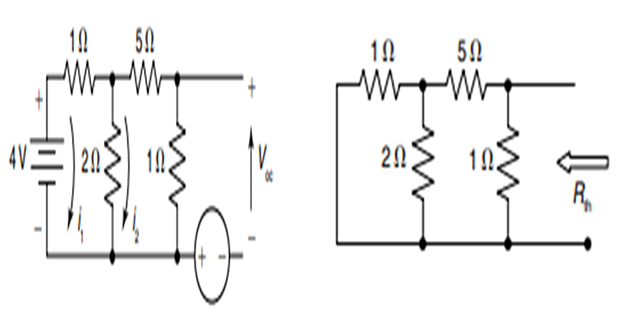
\[\begin{aligned} \text{Mesh-1}~3i_1-2i_2 & = 4\\ \text{Mesh-2}~8i_2-2i_1 & = 0\\ \text{solving}~i_2 & = \dfrac{2}{5}~\mathrm{A} \\ \because 1\times i_2 + 6 & = V_{oc} \\ \Rightarrow V_{oc} & = \left(6+\dfrac{2}{5}\right)\\ & =\dfrac{32}{5}~\mathrm{V} \end{aligned}\] \[\begin{aligned} R_{th}&=\dfrac{17}{20}~\Omega\\ P_{max} & = \dfrac{V_{th}^2}{4R_L} = 12~\mathrm{W} \end{aligned}\]